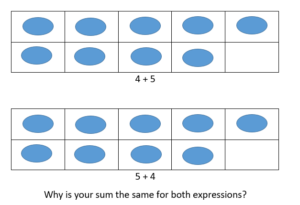
Apply properties of operations as strategies to add and subtract. Example: If 8 + 3 = 11 is known, then 3 + 8 = 11 is also known. (Commutative property of addition)
Cognitive Complexity Level: 1-Recall
[divider] [/divider] Students are able to…
- represent addition and subtraction examples
- describe patterns when adding and subtracting:
- the order does not change the total
- result of adding/subtracting zero and one
- grouping numbers can make the problem easier to solve
- explain their thinking and record equations to match their model
[divider] [/divider] Students are able to…because teachers:
- give opportunities to explore the order of addends
- focus discussions on student observations of patterns rather than teacher explanation
- asks questions to support observations of patterns
[divider] [/divider] Questions to ask students:
- Ask: Why is any is any number minus zero the same as the start number?
- Sample answer that indicates understanding: When you subtract zero, the number doesn’t change because zero means there is nothing to take away.
- Ask: Why is the sum 8 when you build 5 + 3 and when you build 3 + 8?
- Sample answer that indicates understanding: Even if you change the order of the addends, the sum does not change because you still have the same amount. When you start at 5 and count on 3 you get 8 and when you start at 3 and count on 5 you get 8.
[divider] [/divider] Additional Resources:
Additional in depth content knowledge
The Math Learning Center: Number Frames and Number Lines
The Math Learning Center: Number Frames; Number Rack
Brain Pop: Making Ten (free version)
[divider] [/divider] Sample Formative Assessment Tasks:
[divider] [/divider] Resources/Tasks to Support Your Child at Home:
Watch this short video to learn more about the Commutative Property of Addition.
Play “Turn Around Facts” with dice or playing cards (excluding face cards, Aces have value of 1). Deal two cards or roll two dice and write two equations (ex: 6 + 3 = 9 and 3 + 6 = 9). Discuss why switching the order of the addends may be helpful. Possible student answer: “It’s easier to put 6 in my head and count on 3 because 3 is a lesser number. Putting 3 in my head and counting on 6 would take longer.”