What is the associative property of multiplication?
The associative property of multiplication is “an extension of the commutative property; to change the order and group two factors to find convenient products (such as 10) in order to make the multiplication easier. Students may begin to use parantheses as well at this level.”
– The Common Core Mathematics Companion: The Standards Decoded, Grades 3-5
3rd grade students were exposed to the associative property in 2nd grade with addition. They learned that when needing to solve 3 + 25 + 17 + 15, they can rearrange the addends to (3 + 17) + (25 + 15). They can easily compute 3 + 17 = 20, 25 + 15 = 40, then 20 + 40 = 60.
In 3rd grade students should be exposed to the associative property of multiplication in context, using the same numbers. For example, “Each week, Kelly works 2 days for 4 hours each day and earns $5 an hour. Len works 5 days for 2 hours each day and earns $4 an hour.”
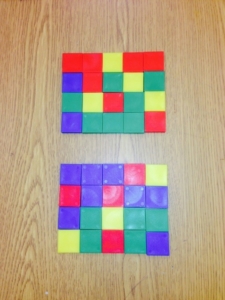
The problems could be modeled using square tile arrays, as shown below.
Model A – Each week, Kelly works 2 days for 4 hours each day and earns $5 an hour.
Model B – Len works 5 days for 2 hours each day and earns $4 an hour.
Students could then extend to record expressions that match their models.
• 2 x (4 x 5) – Each week, Kelly works 2 days for 4 hours each day and earns $5 an hour.
• (2 x 4) x 5 – Len works 5 days for 2 hours each day and earns $4 an hour.
Questions that you will want to ask to deepen student understanding of the associative property of multiplication include:
• How do the models relate?
• What’s the same between the two story problems? What’s different?
• How do the actions in the story problems compare?
• How did you determine what would be your groups?
• How did you determine how many rows/columns would be in each group?
What we want students to discover through exploration is that first they have to determine what represents the overall groups. Then from there, have students determine what represents the smaller groups and how many in each group. Having students contextualize and decontextualize the problems will help deepen their understanding of the associative property. We also want students to realize through exploration, that the product is the same no matter the order of the factors. It’s the model that looks different.
For Example in the above problem for Kelly – the days represented the larger groups, the hours represented the smaller groups, and the dollars represent how many in each of the smaller groups.
Also in 3rd grade, students could look at an expression, such as 3 x 8 x 5, and determine which two factors they want to multiply first to solve efficiently. A student may choose 3 x (8 x 5), because 8 x 5 leads to a product that is a multiple of ten and easier to multiply. So 8 x 5 = 40, then multiply 40 x 3 to get 120.
It’s important to know that students will not be assessed on FSA in context, within a story problem. It is still important to introduce this property in context, for understanding, and encourage students to explore and discover how the property works. See the item specification for the standard involving the associative property of multiplication below: